Theory of differential offset continuation

Bibliography

Babich, V. M., 1991, Short-wavelength diffraction theory: asymptotic methods: Springer-Verlag.

Bagaini, C., and U. Spagnolini, 1993, Common shot velocity analysis by shot continuation operator: 63rd Ann. Internat. Mtg, Soc. of Expl. Geophys., 673-676.

----, 1996, 2-D continuation operators and their applications: Geophysics, 61, 1846-1858.

Bale, R., and H. Jakubowicz, 1987, Post-stack prestack migration: 57th Ann. Internat. Mtg, Soc. of Expl. Geophys., Session:S14.1.

Beylkin, G., 1985, Imaging of discontinuities in the inverse scattering problem by inversion of a causal generalized Radon transform: Journal of Mathematical Physics, 26, 99-108.

Biondi, B., and N. Chemingui, 1994, Transformation of 3-D prestack data by azimuth moveout (AmO): 64th Ann. Internat. Mtg, Soc. of Expl. Geophys., 1541-1544.

Biondi, B., S. Fomel, and N. Chemingui, 1998, Azimuth moveout for 3-D prestack imaging: Geophysics, 63, 574-588.

Black, J. L., K. L. Schleicher, and L. Zhang, 1993, True-amplitude imaging and dip moveout: Geophysics, 58, 47-66.

Bleistein, N., 1984, Mathematical methods for wave phenomena: Academic Press Inc. (Harcourt Brace Jovanovich Publishers).

----, 1990, Born DMO revisited, in 60th Annual Internat. Mtg., Soc. Expl. Geophys., Expanded Abstracts: Soc. Expl. Geophys., 1366-1369.

Bleistein, N., and H. H. Jaramillo, 2000, A platform for Kirchhoff data mapping in sealer models of data acquisition: Geophys. Prosp., 48, 135-162.

Bleistein, N., J. W. Stockwell, and J. K. Cohen, 2001, Mathematics of multidimensional seismic imaging, migration, and inversion: Springer Verlag.

Bolondi, G., E. Loinger, and F. Rocca, 1982, Offset continuation of seismic sections: Geophys. Prosp., 30, 813-828.

Canning, A., and G. H. F. Gardner, 1996, Regularizing 3-D data sets with DmO: Geophysics, 61, 1103-1114.

(Discussion and reply in GEO-62-4-1331).

Chemingui, N., and B. Biondi, 1996, Handling the irregular geometry in wide-azimuth surveys: 66th Ann. Internat. Mtg, Soc. of Expl. Geophys., 32-35.

Claerbout, J. F., 1976, Fundamentals of geophysical data processing: Blackwell.

Courant, R., 1962, Methods of mathematical physics: Interscience Publishers.

Deregowski, S. M., and F. Rocca, 1981, Geometrical optics and wave theory of constant offset sections in layered media: Geophys. Prosp., 29, 374-406.

Fomel, S., 2001, Three-dimensional seismic data regularization: PhD thesis, Stanford University.

----, 2003a, Asymptotic pseudounitary stacking operators: Geophysics, 68, 1032-1042.

----, 2003b, Seismic reflection data interpolation with differential offset and shot continuation: Geophysics, 68, 733-744.

Fomel, S., and N. Bleistein, 2001, Amplitude preservation for offset continuation: Confirmation for Kirchhoff data: Journal of Seismic Exploration, 10, 121-130.

Fomel, S., N. Bleistein, H. Jaramillo, and J. K. Cohen, 1996, True amplitude DmO, offset continuation and AvA/AvO for curved reflectors: 66th Ann. Internat. Mtg, Soc. of Expl. Geophys., 1731-1734.

Fomel, S. B., 1994, Kinematically equivalent differential operator for offset continuation of seismic sections: Russian Geology and Geophysics, 35, 122-134.

Fomel, S. B., and B. L. Biondi, 1995, The time and space formulation of azimuth moveout: 65th Ann. Internat. Mtg, Soc. of Expl. Geophys., 1449-1452.

Gazdag, J., 1978, Wave equation migration with the phase-shift method: Geophysics, 43, 1342-1351.

Goldin, S., 1990, A geometric approach to seismic processing: the method of discontinuities, in SEP-67: Stanford Exploration Project, 171-210.

Goldin, S. V., 1988, Transformation and recovery of discontinuities in problems of tomographic type: Institute of Geology and Geophysics.

----, 1994, Superposition and continuation of tranformations used in seismic migration: Russian Geology and Geophysics, 35, 131-145.

Goldin, S. V., and S. B. Fomel, 1995, Estimation of reflection coefficient in DMO: Russian Geology and Geophysics, 36, 103-115.

Gradshtein, I. S., and I. M. Ryzhik, 1994, Table of integrals, series, and products: Boston: Academic Press.

Haddon, R. A. W., and P. W. Buchen, 1981, Use of Kirchhoff's formula for body wave calculations in the earth: Geophys. J. Roy. Astr. Soc., 67, 587-598.

Hale, D., 1984, Dip-moveout by Fourier transform: Geophysics, 49, 741-757.

----, 1991, Course notes: Dip moveout processing: Soc. Expl. Geophys.

----, 1995, DMo Processing, in DMO processing: Soc. of Expl. Geophys., 496.

(Reprints).

Hale, I. D., 1983, Dip moveout by Fourier transform: PhD thesis, Stanford University.

Hill, S. J., R. Stolt, and S. Chiu, 2001, Altering offsets and azimuths: The Leading Edge, 20, 210-213.

Liner, C., 1990, General theory and comparative anatomy of dip moveout: Geophysics, 55, 595-607.

Liner, C. L., 1991, Born theory of wave-equation dip moveout: Geophysics, 56, 182-189.

Liner, C. L., and J. K. Cohen, 1988, An amplitude-preserving inverse of hale`s DmO: 58th Ann. Internat. Mtg, Soc. of Expl. Geophys., Session:S17.5.

Notfors, C. D., and R. J. Godfrey, 1987, Dip moveout in the frequency-wavenumber domain (short note): Geophysics, 52, 1718-1721.

Petkovsek, M., H. S. Wilf, and D. Zeilberger, 1996, $A=B$: A K Peters Ltd.

Ronen, J., 1987, Wave equation trace interpolation: Geophysics, 52, 973-984.

Ronen, S., 1994, Handling irregular geometry: Equalized DmO and beyond: 64th Ann. Internat. Mtg, Soc. of Expl. Geophys., 1545-1548.

Ronen, S., V. Sorin, and R. Bale, 1991, Spatial dealiasing of 3-D seismic reflection data: Geophysical Journal International, 503-511.

Stolt, R. H., 1978, Migration by Fourier transform: Geophysics, 43, 23-48.

(Discussion and reply in GEO-60-5-1583).

Stovas, A. M., and S. B. Fomel, 1996, Kinematically equivalent integral DMO operators: Russian Geology and Geophysics, 37, 102-113.

Tenenbaum, M., and H. Pollard, 1985, Ordinary differential equations : an elementary textbook for students of mathematics, engineering, and the sciences: Dover Publications.

Cervený, V., 2001, Seismic ray theory: Cambridge University Press.

Watson, G. N., 1952, A treatise on the theory of Bessel functions, 2nd ed.: Cambridge University Press.

Zhou, B., I. M. Mason, and S. A. Greenhalgh, 1996, An accurate formulation of log-stretch dip moveout in the frequency-wavenumber domain: Geophysics, 61, 815-820.

Appendix A

Second-order reflection traveltime derivatives

This appendix contains a derivation of equations connecting second-order partial derivatives of the reflection traveltime with the geometric properties of the reflector in a constant velocity medium. These equations are used in the main text of this paper to describe the amplitude behavior of offset continuation. Let $\tau(s,r)$ be the reflection traveltime from the source $s$ to the receiver $r$. Consider a formal equality

$$\tau(s,r)=\tau_1\left(s,x(s,r)\right)+\tau_2\left(x(s,r),r\right)\;,$$ (114)

where $x$ is the reflection point parameter, $\tau_1$ corresponds to the incident ray, and $\tau_2$ corresponds to the reflected ray. Differentiating (A-1) with respect to $s$ and $r$ yields

$${\partial \tau \over \partial s} = {\partial \tau_1 \over \partial s} + {\partial \tau \over \partial x}\, {\partial x \over \partial s}\;,$$ (115)

$${\partial \tau \over \partial r} = {\partial \tau_2 \over \partial r} + {\partial \tau \over \partial x}\, {\partial x \over \partial r}\;.$$ (116)

According to Fermat's principle, the two-point reflection ray path must correspond to the traveltime stationary point. Therefore

$${\partial \tau \over \partial x} \equiv 0$$ (117)

for any $s$ and $r$. Taking into account (A-4) while differentiating (A-2) and (A-3), we get

$${\partial^2 \tau \over \partial s^2} = {\partial^2 \tau_1 \over \partial s^2} + B_1\, {\partial x \over \partial s}\;,$$ (118)

$${\partial^2 \tau \over \partial r^2} = {\partial^2 \tau_2 \over \partial r^2} + B_2\, {\partial x \over \partial r}\;,$$ (119)

$${\partial^2 \tau \over \partial s \partial r} = B_1\, {\partial x \over \partial r}\;= B_2\, {\partial x \over \partial s}\;,$$ (120)

where

$$B_1={\partial^2 \tau_1 \over \partial s \partial x}\;;\; B_2={\partial^2 \tau_2 \over \partial r \partial x}\;.$$

Differentiating equation (A-4) gives us the additional pair of equations

$$C\,{\partial x \over \partial s}+B_1 = 0\;,$$ (121)

$$C\,{\partial x \over \partial r}+B_2 = 0\;,$$ (122)

where

$$C={\partial^2 \tau \over \partial x^2}= {\partial^2 \tau_1 \over \partial x^2}+ {\partial^2 \tau_2 \over \partial x^2}\;.$$

Solving the system (A-8) - (A-9) for $\partial x \over \partial s$ and $\partial x \over \partial r$ and substituting the result into (A-5) - (A-7) produces the following set of expressions:

$${\partial^2 \tau \over \partial s^2} = {\partial^2 \tau_1 \over \partial s^2} - C^{-1}\,B_1^2\;;$$ (123)

$${\partial^2 \tau \over \partial r^2} = {\partial^2 \tau_2 \over \partial r^2} - C^{-1}\,B_2^2\;;$$ (124)

$${\partial^2 \tau \over \partial s \partial r} = - C^{-1}\,B_1\,B_2\;.$$ (125)

In the case of a constant velocity medium, expressions (A-10) to (A-12) can be applied directly to the explicit equation for the two-point eikonal

$$\tau_1(y,x)=\tau_2(x,y)={\sqrt{(x-y)^2+z^2(x)}\over v}\;.$$ (126)

Differentiating (A-13) and taking into account the trigonometric relationships for the incident and reflected rays (Figure 1), one can evaluate all the quantities in (A-10) to (A-12) explicitly. After some heavy algebra, the resultant expressions for the traveltime derivatives take the form

$${\partial \tau \over \partial s} = {\partial \tau_1 \over \partial s} = {\sin{\alpha_1}\over v} \;;\; {\partial \tau \over \partial r} = {\partial \tau_2 \over \partial r} = {\sin{\alpha_2}\over v}\;;$$ (127)

$${\partial \tau_1 \over \partial x} = {\sin{\gamma}\over v \cos{\alpha}} \;;\; {\partial \tau_2 \over \partial x} = - {\sin{\gamma}\over v \cos{\alpha}}\;;$$ (128)

$$B_1 = {\partial^2 \tau_1 \over \partial s\,\partial x} = {\cos{\alpha_1... ...{\alpha}}}\, \left(-1-{\sin{\gamma}\over\cos{\alpha}}\,\sin{\alpha_1}\right)\;;$$ (129)

$$B_2 = {\partial^2 \tau_2 \over \partial r\,\partial x} = {\cos{\alpha_2... ...{\alpha}}}\, \left(-1+{\sin{\gamma}\over\cos{\alpha}}\,\sin{\alpha_2}\right)\;;$$ (130)

$$B_1\,B_2 = {\cos^6{\gamma}\over v^2\,D^2\,a^4}\;;\; B_1+B_2 = -2\,{\cos^3{\gamma}\over v\,D\,a^2}\,\left(2\,a^2-1\right)\;;$$ (131)

$${\partial^2 \tau_1 \over \partial x^2} = {{\cos^2{\gamma}+D\... ...2{\gamma}+D\,K}\over{v\,D\,\cos^3{\alpha}}}\,\cos{\alpha_2}\;;$$ (132)

$$C={\partial^2 \tau_1 \over \partial x^2}+{\partial^2 \tau_2 ... ...\gamma}\,{{\cos^2{\gamma}+D\,K}\over{v\,D\,\cos^3{\alpha}}}\;.$$ (133)

Here $D$ is the length of the normal (central) ray, $\alpha$ is its dip angle ( $\alpha={{\alpha_1+\alpha_2}\over 2}$, $\tan{\alpha}=z'(x)$), $\gamma$ is the reflection angle $\left(\gamma={{\alpha_2-\alpha_1}\over 2}\right)$, $K$ is the reflector curvature at the reflection point $\left(K=z''(x)\,\cos^3{\alpha}\right)$, and $a$ is the dimensionless function of $\alpha$ and $\gamma$ defined in (47).

The equations derived in this appendix were used to obtain the equation

$$\tau_n\,\left({\partial^2 \tau_n \over \partial y^2}- {\part... ...\,\left({\sin^2{\alpha}+DK}\over {\cos^2{\gamma}+DK}\right)\;,$$ (134)

which coincides with (50) in the main text.

Appendix B

The kinematics of offset continuation

This Appendix presents an alternative method to derive equation (70), which describes the summation path of the integral offset continuation operator. The method is based on the following considerations.

The summation path of an integral (stacking) operator coincides with the phase function of the impulse response of the inverse operator. Impulse response is by definition the operator reaction to an impulse in the input data. For the case of offset continuation, the input is a reflection common-offset gather. From the physical point of view, an impulse in this type of data corresponds to the special focusing reflector (elliptical isochrone) at the depth. Therefore, reflection from this reflector at a different constant offset corresponds to the impulse response of the OC operator. In other words, we can view offset continuation as the result of cascading prestack common-offset migration, which produces the elliptic surface, and common-offset modeling (inverse migration) for different offsets. This approach resemble that of Deregowski and Rocca (1981). It was also applied to a more general case of azimuth moveout (AMO) by Fomel and Biondi (1995) and fully generalized by Bleistein and Jaramillo (2000). The geometric approach implies that in order to find the summation pass of the OC operator, one should solve the kinematic problem of reflection from an elliptic reflector whose focuses are in the shot and receiver locations of the output seismic gather.

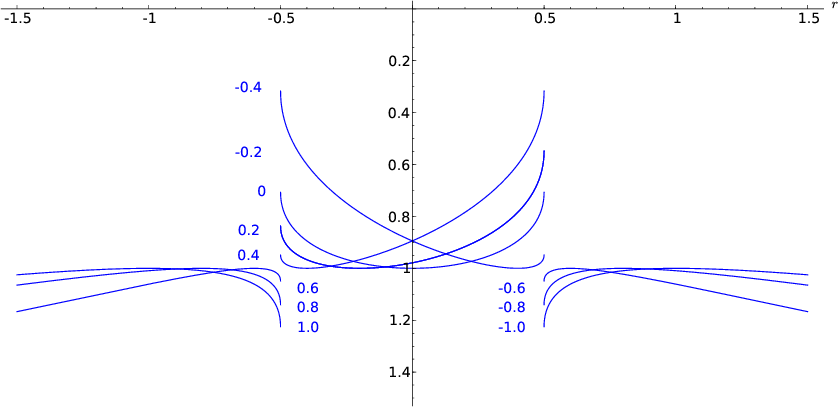
In order to solve this problem , let us consider an elliptic surface of the general form

$$h(x)=\sqrt{d^2-\beta\,(x-x')^2}\;,$$ (135)

where $0 < \beta < 1$. In a constant velocity medium, the reflection ray path for a given source-receiver pair on the surface is controlled by the position of the reflection point $x$. Fermat's principle provides a required constraint for finding this position. According to Fermat's principle, the reflection ray path corresponds to a stationary value of the travel-time. Therefore, in the neighborhood of this path,

$${\partial \tau(s,r,x) \over \partial x} = 0\;,$$ (136)

where $s$ and $r$ stand for the source and receiver locations on the surface, and $\tau$ is the reflection traveltime

$$\tau(s,r,x) = { \sqrt{h^2(x)+(s-x)^2} \over v} + { \sqrt{h^2(x)+(r-x)^2} \over v}\;.$$ (137)

Substituting equations (B-3) and (B-1) into (B-2) leads to a quadratic algebraic equation on the reflection point parameter $x$. This equation has the explicit solution

$$x(s,r)= x' + {{\xi^2+H^2-h^2+\mbox{sign}(h^2-H^2)\, \sqrt{\l... ...^2-H^2-h^2\right)^2-4\,H^2\,h^2}\over {2\,\xi\,(1-\beta)}}}\;,$$ (138)

where $h=(r-s)/2$, $\xi = y-x'$, $y=(s+r)/2$, and $H^2=d^2\,\left({1 \over \beta} - 1\right)$. Replacing $x$ in equation (B-3) with its expression (B-4) solves the kinematic part of the problem, producing the explicit traveltime expression

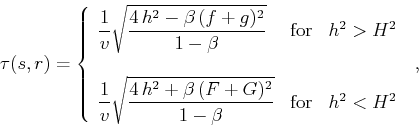$$\tau(s,r)=\left\{ \begin{array}{lcr}\displaystyle{ {1 \over... ... {1-\beta}}} & \mbox{for} & h^2 < H^2 \end{array} \right.\;,$$ (139)

where

$$f=\sqrt{(r-x')^2-H^2}\; , \;g=\sqrt{(s-x')^2-H^2}\;,$$

$$F=\sqrt{H^2-(r-x')^2}\; , \;G=\sqrt{H^2-(s-x')^2}\;.$$

The two branches of equation (B-5) correspond to the difference in the geometry of the reflected rays in two different situations. When a source-and-receiver pair is inside the focuses of the elliptic reflector, the midpoint $y$ and the reflection point $x$ are on the same side of the ellipse with respect to its small semi-axis. They are on different sides in the opposite case (Figure B-1).

ell Figure B-1. .

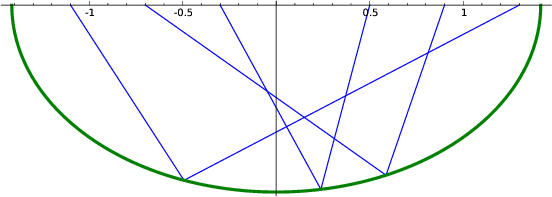
Reflections from an ellipse. The three pairs of reflected rays correspond to a common midpoint (at 0.1) and different offsets. The focuses of the ellipse are at 1 and -1.

If we apply the NMO correction, equation (B-5) is transformed to

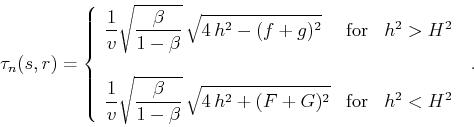$$\tau_n(s,r)=\left\{ \begin{array}{lcr}\displaystyle{ {1 \ov... ...^2+(F+G)^2}} & \mbox{for} & h^2 < H^2 \end{array} \right.\;.$$ (140)

Then, recalling the relationships between the parameters of the focusing ellipse $r$, $x'$ and $\beta$ and the parameters of the output seismic gather (Deregowski and Rocca, 1981)

$$r={ {v\,t_n} \over 2}\;,\;x'=y\;,\; \beta={t_n^2 \over {t_n^2+{{4\,h^2} \over v^2}}}\;,\; H=h\;,$$ (141)

and substituting expressions (B-7) into equation (B-6) yields the expression

$$t_1(s_1,r_1;s,r,t_n)=\left\{ \begin{array}{lcr}\displaystyl... ...+(F+G)^2}} & \mbox{for} & h_1^2 < h^2 \end{array} \right.\;,$$ (142)

where

$$f=\sqrt{(r_1-r)\,(r_1-s)}\;,\;g=\sqrt{(s_1-r)\,(s_1-s)}\;,$$

$$F=\sqrt{(r-r_1)\,(r_1-s)}\;,\;G=\sqrt{(s_1-r)\,(s-s_1)}\;.$$

It is easy to verify algebraically the mathematical equivalence of equation (B-8) and equation (70) in the main text. The kinematic approach described in this appendix applies equally well to different acquisition configurations of the input and output data. The source-receiver parameterization used in (B-8) is the actual definition for the summation path of the integral shot continuation operator (Bagaini and Spagnolini, 1996,1993). A family of these summation curves is shown in Figure B-2.

shc
Figure B-2. .

Summation paths of the integral shot continuation. The output source is at -0.5 km. The output receiver is at 0.5 km. The indexes of the curves correspond to the input source location.

Theory of differential offset continuation