 |
 |
 |
 | Asymptotic pseudounitary stacking operators |  |
![[pdf]](icons/pdf.png) |
Next: 3. Common-offset migration
Up: Migration
Previous: 1. Common-shot migration
In the case of zero-offset migration, Gritsenko's formula
simplifies the true-amplitude migration weighting function
(46) to the form
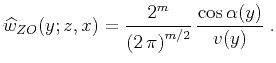 |
(49) |
In a constant-velocity medium, one can accomplish the true-amplitude
zero-offset migration by premultiplying the recorded zero-offset
seismic section by the factor
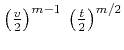 [which corresponds at the stationary point to the
geometric spreading
[which corresponds at the stationary point to the
geometric spreading 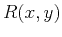 ] and downward continuation according to
formula (40) with the effective velocity
] and downward continuation according to
formula (40) with the effective velocity  (Hubral et al., 1991; Goldin, 1987). This conclusion is in
agreement with the analogous result of Born inversion
(Bleistein et al., 1985), though derived from a different viewpoint.
(Hubral et al., 1991; Goldin, 1987). This conclusion is in
agreement with the analogous result of Born inversion
(Bleistein et al., 1985), though derived from a different viewpoint.
In the zero-offset case, the pseudo-unitary forward operator reduces to
downward pseudo-unitary continuation with a velocity of  .
.
2013-03-03
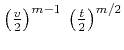 [which corresponds at the stationary point to the
geometric spreading
[which corresponds at the stationary point to the
geometric spreading ![]() .
.