Theory of differential offset continuation

Comparison with Bolondi's OC equation

Equation (1) and the previously published OC equation (Bolondi et al., 1982) differ only with respect to the single term $\partial^2 P \over {\partial h^2}$. However, this difference is substantial.

From the offset continuation characteristic equation (4), we can conclude that the first-order traveltime derivative with respect to offset decreases with decreasing offset. The derivative equals zero at the zero offset, as predicted by the principle of reciprocity (the reflection traveltime has to be an even function of offset). Neglecting $\left({\partial \tau_n} \over {\partial h}\right)^2$ in (4) leads to the characteristic equation

$$h \, {\left( \partial \tau_n \over \partial y \right)}^2 = \, - \, \tau_n \, {\partial \tau_n \over \partial h}\;,$$ (13)

which corresponds to the approximate OC equation of Bolondi et al. (1982). The approximate equation has the form

$$h \, {\partial^2 P \over \partial y^2} \, = \, t_n \, {\partial^2 P \over {\partial t_n \, \partial h}}\;.$$ (14)

Comparing equations (13) and (4), we can note that approximation (13) is valid only if

$${\left( \partial \tau_n \over \partial h \right)}^2 \, \ll\, {\left( \partial \tau_n \over \partial y \right)}^2 \,\,\,.$$ (15)

To find the geometric constraints implied by inequality (15), we can express the traveltime derivatives in geometric terms. As follows from expressions (10) and (11),

$${{\partial \tau} \over {\partial y}} = {{\partial \tau} \over {\partial r}} + {{\partial \tau} \over {\partial s}} \,=\, { {2 \sin{\alpha} \cos{\gamma}} \over {v}}\;,$$ (16)

$${{\partial \tau} \over {\partial h}} = {{\partial \tau} \over {\partial r}} - {{\partial \tau} \over {\partial s}} \,=\, { {2 \cos{\alpha} \sin{\gamma}} \over {v}}\;.$$ (17)

Expression (9) allows transforming equations (16) and (17) to the form

$$\tau_n \, {{\partial \tau_n} \over {\partial y}} = \tau \, {{\partial \tau} \over {\partial y}} \,=\, 4h\,{{\sin{\alpha} \cos{\alpha} \cot{\gamma}} \over {v^2}}\;;$$ (18)

$$\tau_n \, {{\partial \tau_n} \over {\partial h}} = \tau \, {{\partial \tau} \over {\partial h}} - {{4h} \over {v^2}} \,=\,-\, 4h\,{{\sin^2{\alpha}} \over {v^2}}\;.$$ (19)

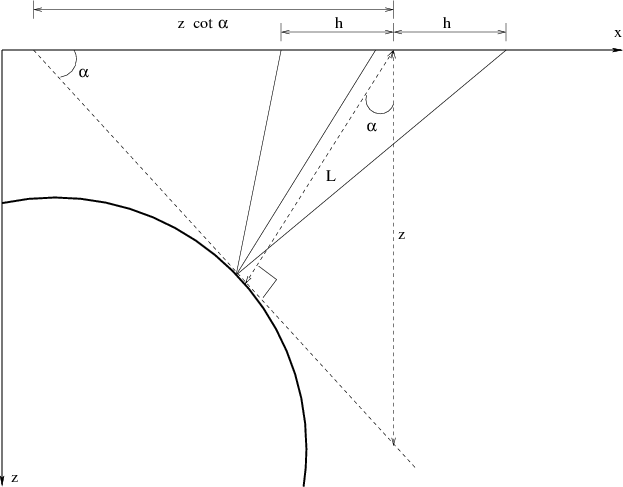
Without loss of generality, we can assume $\alpha$ to be positive. Consider a plane tangent to a true reflector at the reflection point (Figure 2). The traveltime of a wave, reflected from the plane, has the known explicit expression

$$\tau\,=\,{2 \over v}\,\sqrt{L^2+h^2\,\cos^2{\alpha}}\,\,\,,$$ (20)

where $L$ is the length of the normal ray from the midpoint. As follows from combining (20) and (9),

$${\cos{\alpha} \cot{\gamma}} \,=\, {L \over h} \,\,\,.$$ (21)

We can now combine equations (21), (18), and (19) to transform inequality (15) to the form

$$h \ll {L \over {\sin{\alpha}}} \,=\, z\, \cot{\alpha}\,\,,$$ (22)

where $z$ is the depth of the plane reflector under the midpoint. For example, for a dip of 45 degrees, equation (14) will be satisfied only for offsets that are much smaller than the depth of the reflector.

ocobol Figure 2. Reflection rays and tangent to the reflector in a constant velocity medium (a scheme).

Theory of differential offset continuation