-
Aki, K., and P. Richards, 2002, Quantitative seismology (second edition):
University Science Books.
-
-
Barnes, A. E., 1991, Instantaneous frequency and amplitude at the envelope
peak of a constant-phase wavelet: Geophysics, 56, 1058-1060.
-
-
Bracewell, R., 1978, The fourier transform and its applications, 2nd ed.:
McGraw-HillInc.
-
-
Brzostowski, M., and G. McMechan, 1992, 3-D tomographic imaging of
near-surface seismic velocity and attenuation: Geophysics, 57,
396-403.
-
-
Canales, L., 1984, Random noise reduction: 54th Annual International Meeting,
SEG, Expanded Abstracts, 525-527.
-
-
Carcione, J. M., 2007, Wave fields in real media: Wave propagation in
anisotropic, anelastic, porous and electromagnetic media: Elsevier.
-
-
Carcione, J. M., F. Cavallini, F. Mainardi, and A. Hanyga, 2002, Time-domain
modeling of constant-Q seismic waves using fractional derivatives: Pure and
Applied Geophysics, 159, 1719-1736.
-
-
Carmona, R., W. Hwang, and B. Torresani, 1998, Practical time-frequency
analysis: Gabor and wavelet transforms with an implementation is s: Academic
Press.
-
-
Chen, Y., 2017, Fast dictionary learning for noise attenuation of
multidimensional seismic data: Geophysical Journal International, 209,
no. 1, 21-31.
-
-
Chen, Y., and S. Fomel, 2015, Random noise attenuation using local
signal-and-noise orthogonalization: Geophysics, 80, WD1-Wd9.
-
-
Chen, Y., D. Zhang, Z. Jin, X. Chen, S. Zu, W. Huang, and S. Gan, 2016,
Simultaneous denoising and reconstruction of 5D seismic data via damped
rank-reduction method: Geophysical Journal International, 206, no. 3,
1695-1717.
-
-
Cheng, P., and G. F. Margrave, 2012, Q estimation by a match-filter method:
SEG expanded abstracts: 82nd Annual international meeting, 1-6.
-
-
Delle Piane, C., J. Sarout, C. Madonna, E. H. Saenger, D. N. Dewhurst, and M.
Raven, 2014, Frequency-dependent seismic attenuation in shales: experimental
results and theoretical analysis: Geophysical Journal International, 198, 504-515.
-
-
Du, J., S. Lin, W. Sun, and G. Liu, 2010, Seismic attenuation estimation using
s transform with regularized inversion: 80th Annual International Meeting,
SEG, Expanded Abstracts, 2901-2904.
-
-
Dutta, G., and G. T. Schuster, 2014, Attenuation compensation for
least-squares reverse time migration using the viscoacoustic-wave equation:
Geophysics, 79, S251-S262.
-
-
Engelhard, L., 1996, Determination of seismic-wave attenuation by complex
trace analysis: Geophysical Journal International, 125, 608-622.
-
-
Fomel, S., 2007a, Local seismic attributes: Geophysics, 72, A29-A33.
-
-
----, 2007b, Shaping regularization in geophysical-estimation problems:
Geophysics, 72, R29-R36.
-
-
Futterman, W. I., 1962, Dispersive body waves: Journal of Geophysical
Research, 67, 5279-5291.
-
-
Gan, S., S. Wang, Y. Chen, S. Qu, and S. Zu, 2016, Velocity analysis of
simultaneous-source data using high-resolution semblance-coping with the
strong noise: Geophysical Journal International, 204, 768-779.
-
-
Gao, J., and S. Yang, 2007, On the method of quality factors estimation from
zero-offset vsp data: Chines Journal of Geophysics, 50, 1026-1040.
-
-
Guo, R., G. Yu, and S. Wang, 2014, A novel method for Q estimating: Beijing
2014 International Geophysical Conference & Exposition, 377-381.
-
-
Hauge, P. S., 1981, Measurements of attenuation from vertical seismic
profiles: Geophysics, 46, 1548-1558.
-
-
Hedlin, K., L. Mewhort, and G. Margrave, 2001, Delineation of steam flood
using seismic attenuation: SEG expanded abstracts: 71st Annual international
meeting, 1572-1575.
-
-
Jannsen, D., J. Voss, and F. Theilen, 1985, Comparison of methods to determine
q in shallow marine sediments from vertical reflection seismograms:
Geophysical Prospecting, 33, 479-497.
-
-
Kjartansson, E., 1979, Constant Q-wave propagation and attenuation: Journal
of Geophysical Research Solid Earth, 84, 4737-4748.
-
-
Kneib, G., and S. A. Shapiro, 1995, Viscoacoustic wave propagation in 2-d
random media and separation of absorption and scattering attenuation:
Geophysics, 60, 459-467.
-
-
Lawrence, J. F., P. M. Shearer, and G. Masters, 2006, Mapping attenuation
beneath north america using waveform cross-correlation and cluster analysis:
Geophysical Research Letters, 33, L07315.
-
-
Li, H., W. Zhao, H. Zhao, F. Yao, and L. Shao, 2006, Measures of scale based
on the wavelet scalogram with applications to seismic attenuation:
Geophysics, 71, V111-V118.
-
-
Li, J., S. Wang, D. Yang, G. Tang, and Y. Chen, 2018, Subsurface attenuation
estimation using a novel hybrid method based on fwe function and power
spectrum: Exploration Geophysics, 49, no. 2, 220-230.
-
-
Li, Q., H. Zhou, Q. Zhang, H. Chen, and S. Sheng, 2015, Efficient reverse time
migration based on fractional laplacian viscoacoustic wave equation:
Geophysical Journal International, 204, 488-504.
-
-
Matheney, M. P., and R. L. Nowack, 1995, Seismic attenuation values obtained
from instantaneous frequency matching and spectral ratios: Geophysical
Journal International, 123, 1-15.
-
-
Matsushima, J., M. Y. Ali, and F. Bouchaala, 2015, Seismic attenuation
estimation from zero-offset vsp data using seismic interferometry:
Geophysical Journal International, 204, 1288-1307.
-
-
Mousavi, S. M., and C. A. Langston, 2016, Hybrid seismic denoising using
higher-order statistics and improved wavelet block thresholding: Bulletin of
the Seismological Society of America, 106, no. 4, 1380-1393.
-
-
----, 2017, Automatic noise-removal/signal-removal based on general
cross-validation thresholding in synchrosqueezed domain and its application
on earthquake data: Geophysics, 82, no. 4, V211-V227.
-
-
Mousavi, S. M., C. A. Langston, and S. P. Horton, 2016, Automatic microseismic
denoising and onset detection using the synchrosqueezed continuous wavelet
transform: Geophysics, 81, no. 4, V341-V355.
-
-
Prudencio, J., J. M. Ibáñez, A. García-Yeguas, E. Del Pezzo, and
A. M. Posadas, 2013, Spatial distribution of intrinsic and scattering
seismic attenuation in active volcanic islands-ii: Deception island images:
Geophysical Journal International, 195, 1957-1969.
-
-
Quan, Y., and J. M. Harris, 1997, Seismic attenuation tomography using the
frequency shift method: Geophysics, 62, 895-905.
-
-
Raike, S. A., and R. E. White, 1984, Measurements of earth attenuation from
downhole and surface seismic recordings: Geophysical Prospecting, 32,
892-919.
-
-
Raji, W. O., and A. Rietbrock, 2013, Determination of quality factor (q) in
reflection seismic data: SEG expanded abstracts: 83rd Annual international
meeting, 3191-3195.
-
-
Reine, C., M. van der Baan, and R. Clark, 2009, The robustness of seismic
attenuation measurements using fixed- and variable-window time-frequency
transforms: Geophysics, 74, WA123-WA135.
-
-
Ricker, N., 1953, The form and laws of propagation of seismic wavelets:
Geophysics, 18, 10-40.
-
-
Ruan, Y., and Y. Zhou, 2010, The effects of 3-d anelasticity (q) structure on
surface wave phase delays: Geophysical Journal International, 181,
479-492.
-
-
----, 2012, The effects of 3-d anelasticity (q) structure on surface wave
amplitudes: Geophysical Journal International, 189, 967-983.
-
-
Stockwell, R. G., L. Mansinha, and R. P. Lowe, 1996, Localization of the
complex spectrum: The s transform: IEEE Transactions on Signal Processing,
44, 998-1001.
-
-
Tonn, R., 1991, The determination of the seismic quality factor q from vsp
data: A comparison of different computational methods: Geophysical
Prospecting, 39, 1-27.
-
-
Toverud, T., and B. Ursin, 2005, Comparison of seismic attenuation models
using zero-offset vertical seismic profiling (vsp) data: Geophysics, 70, F17-F25.
-
-
Wang, S., D. Yang, J. Li, and H. Song, 2015, Q factor estimation based on the
method of logarithmic spectral area difference: Geophysics, 80,
V157-V171.
-
-
Wang, S.-D., 2011, Attenuation compensation method based on inversion: Applied
Geophysics, 8, 150-157.
-
-
Wang, Y., 2002, A stable and efficient approach of inverse q filtering:
Geophysics, 67, 657-663.
-
-
----, 2004, Q analysis on reflection seismic data: Geophysical Research
Letters, 31, L17606.
-
-
----, 2014, Stable q analysis on vertical seismic profiling data:
Geophysics, 79, D217-D225.
-
-
Wang, Y., X. Ma, H. Zhou, and Y. Chen, 2018a, L1-2 minimization for exact and
stable seismic attenuation compensation: Geophysical Journal International,
213, no. 3, 1629-1646.
-
-
Wang, Y., H. Zhou, H. Chen, and Y. Chen, 2018b, Adaptive stabilization for
Q-compensated reverse time migration: Geophysics, 83, no. 1,
S15-S32.
-
-
Wright, C., and D. Hoy, 1981, A note on pulse broadening and anelastic
attenuation in near-surface rocks: Physics of the Earth and Planetary
interiors, 25, P1-P8.
-
-
Yang, S., J. Gao, W. Chen, D. Wang, and B. Weng, 2009, Comparisons of four
methods used for seismic quality factors estimation: SEG expanded abstracts:
79th Annual international meeting, 2472-2476.
-
-
Zhang, C., and T. J. Ulrych, 2002, Estimation of quality factors from CMP
records: Geophysics, 67, 1542-1547.
-
-
----, 2007, Seismic absorption compensation: A least squares inverse
scheme: Geophysics, 72, R109-R114.
-
-
Zhu, T., 2014, Time-reverse modelling of acoustic wave propagation in
attenuating media: Geophysical Journal International, 197, 483-494.
-
-
----, 2015, Implementation aspects of attenuation compensation in
reverse‐time migration: Geophysical Prospecting, 64, 657-670.
-
-
Zhu, T., and J. Sun, 2017, Viscoelastic reverse time migration with
attenuation compensation: Geophysics, 82, S61-S73.
-
Appendix
A
S transform
For non-stationary data, time-frequency transforms are useful, as they can produce a spectral estimate centered at each time element of the data. In this respect, a 1D data trace is mapped into a 2D spectrogram, which has dimensions of time and frequency (Reine et al., 2009). To introduce the S transform, we first briefly introduce the short-time Fourier transform (STFT).
The STFT is a Fourier-related transform used to determine the sinusoidal frequency and phase content of local sections of a signal as it changes over time
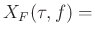 STFT STFT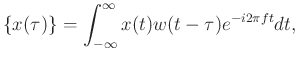 |
(17) |
where  is the frequency,
is the frequency, 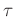 is a parameter that controls the position of the window function along the
is a parameter that controls the position of the window function along the  axis.
axis.
The STFT might be the most recognized time-frequency transform. It can be understood in such way that the data trace  is gated by a sliding window function
is gated by a sliding window function  , and the Fourier transform (Bracewell, 1978). The sliding window function is commonly chosen as a Hanning window or Gaussian window.
, and the Fourier transform (Bracewell, 1978). The sliding window function is commonly chosen as a Hanning window or Gaussian window.
When 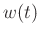 is chosen as a Gaussian window function:
is chosen as a Gaussian window function:
 |
(18) |
where 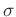 is the distribution width, the STFT transforms to the definition of Gabor transform (Carmona et al., 1998).
is the distribution width, the STFT transforms to the definition of Gabor transform (Carmona et al., 1998).
The S transform was proposed by Stockwell et al. (1996) as an extension to the Morlet wavelet transform. Instead of a fixed time length for each frequency in the window functions chosen for STFT, the S transform analyzes shorter data segments as the frequencies increase. Related with the Gaussian window function as shown in equation A-2, the distribution width 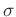 is substituted with:
is substituted with:
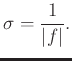 |
(19) |
Besides, the Gaussian window function used in the S transform is normalized with respect to the amplitude. Thus, the width of the Gaussian window scales inversely with frequency and amplitude scales linearly with the frequency:
 |
(20) |
Combining equation A-1 with equation A-4 we can obtain the definition of the S transform (ST):
 ST ST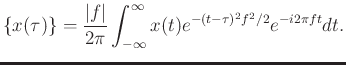 |
(21) |
The S transform use a frequency-dependent window similar to that of wavelet transform, which allows a better resolution of low frequency components and enables a better time resolution of high frequency components.
2020-02-10
![]() is gated by a sliding window function
is gated by a sliding window function ![]() , and the Fourier transform (Bracewell, 1978). The sliding window function is commonly chosen as a Hanning window or Gaussian window.
, and the Fourier transform (Bracewell, 1978). The sliding window function is commonly chosen as a Hanning window or Gaussian window.
![]() is chosen as a Gaussian window function:
is chosen as a Gaussian window function:
![]() is substituted with:
is substituted with: