|
|
|
|
The time and space formulation of azimuth moveout |
Let's consider the general symmetric ellipsoid equation
This section addresses the kinematic problem of reflection from the ellipsoid defined by (15). In particular, we are looking for the answer to the following question: For a given elliptic reflector defined by the input midpoint, offset, and time coordinates, what points on the surface can form a source-receiver pair valid for a reflection? If a point in the output midpoint-offset space cannot be related to a reflection pattern, we should exclude it from the AMO impulse response defined in (1).
Fermat's principle provides a general method of solving the kinematic reflection problems. Consider a formal expression for the two-point
reflection traveltime
Since the reflection point is contained inside the ellipsoid,
its projection obeys the evident inequality
|
|---|
|
amoapp
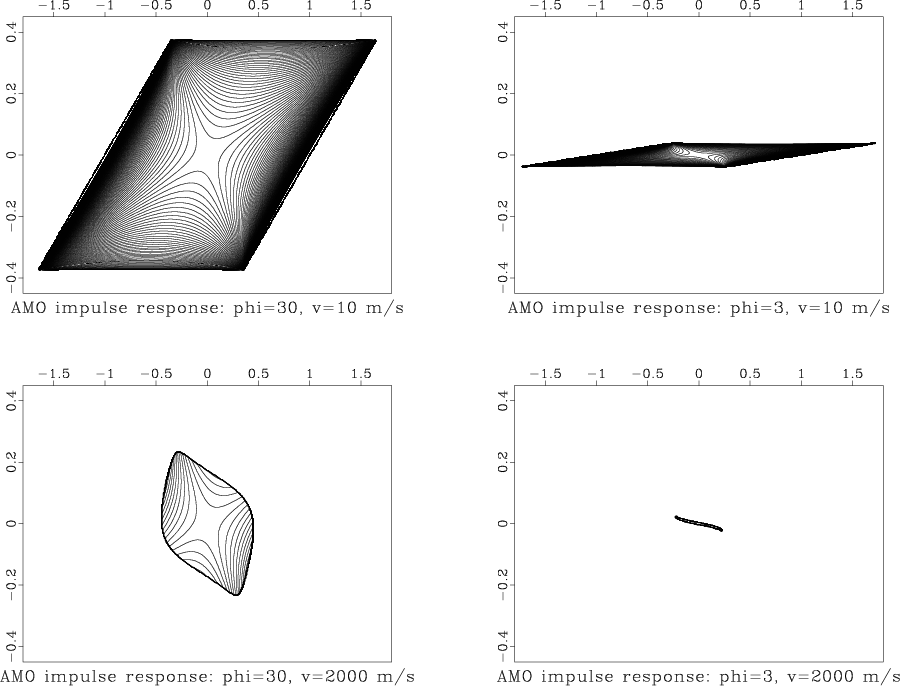
Figure 2. The AMO impulse response traveltime. Parameters: |
|
|
The AMO operator's contours for
different azimuth rotation angles are shown in
Figure 2.
Comparing the results for the case of an unrealistically low velocity
(the top two plots in Figure 2) and the case of a realistic
velocity (the bottom two plots) clearly demonstrates
the gain in the reduction of the aperture size
achieved by the aperture limitation.
The gain is
especially spectacular for small azimuths. When the azimuth rotation
approaches zero, the area of the 3-D aperture monotonously shrinks to a
line, and the limit of the traveltime of the AMO impulse response
(the inverse of (9)) approaches the offset continuation operator
(14) (Figures 3). This means that
taking into account
the aperture limitations of AMO provides a consistent description
valid for small azimuth rotations including zero (the offset
continuation case). Obviously, the cost of an integral operator is
proportional to its size. The size of the offset continuation
operator cannot extend the difference between the offsets
![]() . If we applied DMO and
inverse DMO explicitly, the total size of the two operators would be
about
. If we applied DMO and
inverse DMO explicitly, the total size of the two operators would be
about
![]() , which is
substantially greater. This fact proves that in the case of small
azimuth rotations the AMO price is less than those of not only 3-D prestack
migration, but also 3-D DMO and inverse DMO combined (Canning and Gardner, 1992).
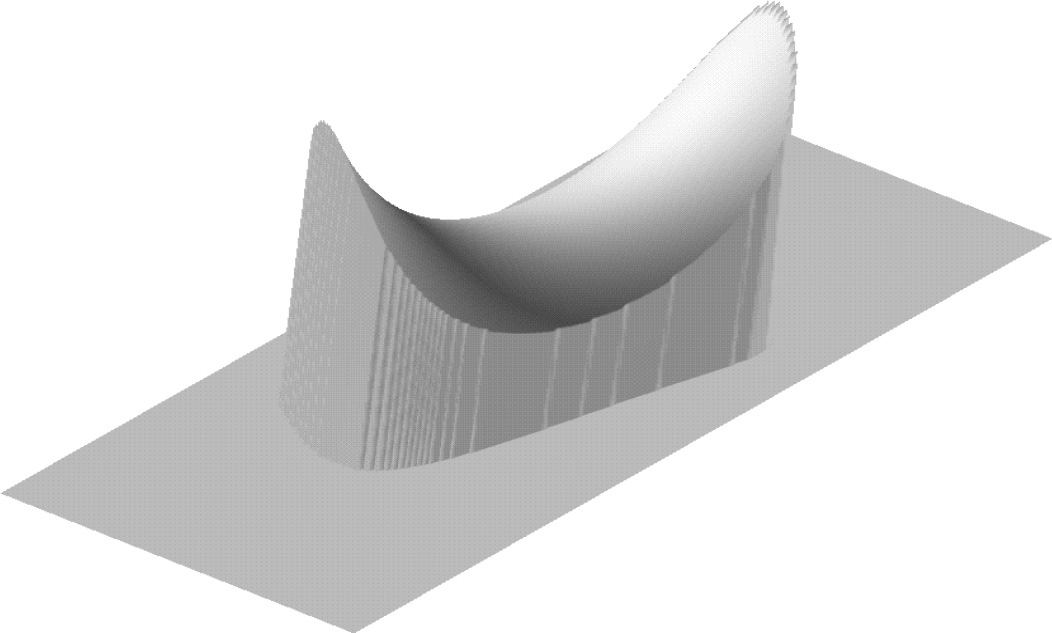
Figure 4 shows the saddle shape of the AMO operator impulse
response in a 3-D AVS display.
, which is
substantially greater. This fact proves that in the case of small
azimuth rotations the AMO price is less than those of not only 3-D prestack
migration, but also 3-D DMO and inverse DMO combined (Canning and Gardner, 1992).
Figure 4 shows the saddle shape of the AMO operator impulse
response in a 3-D AVS display.
|
amocom
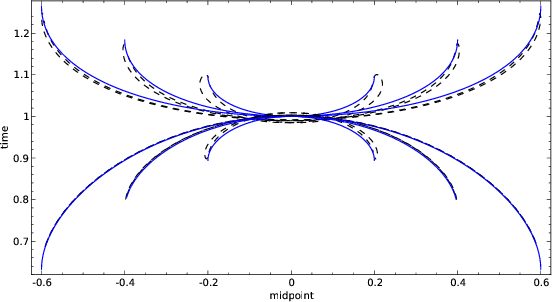
Figure 3. Traveltime curves of the impulse responses. The dashed lines indicate the AMO impulse response with an azimuth rotation of 3 degrees (projection on the |
|
|---|---|
|
|
|
|---|
|
amoavs
Figure 4. AMO impulse response traveltime in three dimensions (the AVS display). Parameters: |
|
|
|
|
|
|
The time and space formulation of azimuth moveout |